Paradox of Triangles

Shadow Gaunt
​Writer, Ravenclaw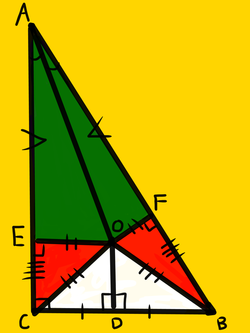

The hypotenuse of a triangle equals its leg. What? That's impossible you say. Well, let me prove you wrong.

We have a triangle here: Triangle ABC. Draw the bisector of angle A and the center perpendicular from BC. They will meet in point O. Thus, the green triangles are equal because they are right triangles. The side AO is shared and the angles OAE and OAF are equal because AO is the bisector. Thus, the green triangles are equal by Hypotenuse-Angle.

The red triangles are equal because they are both right triangles. OE equals OF from the green triangle equality and OC equals OB because OD is the center perpendicular. Thus, the red triangles are equal by Hypotenuse-Leg.

However, we now know that AE=AF and CE=BF, so AE+CE=AF+BF or AC=AB.

So what's the catch? Send your answers to Shadow Gaunt by HOL message. This is worth 10 beans.

Sources: Kvantik Almanah 1

---